Cosmological Revolution III: Tycho and Kepler

A number of problems have been noted with Copernicus' system: (i) the need for epicycles due to Copernicus' continued use of circular orbits, (ii) the lack of new observations to support his system, and (iii) the introduction of the hypothesis of the stars being much further away than previously assumed in order to explain the lack of observed stellar parallax). As a result, very few (probably less than a dozen) individuals adopted his system between the time of his death and the work of Galileo (to be discussed in the next unit). Nonetheless, some interesting developments occured, of which the following are the most important:

Tycho Brahe's Geo-Heliocentric System

Tycho Brahe (1546-1601) attemped to develop a system intermediate between the Ptolemaic (geo-centric) system and the Copernican (helio-centric) system. His system, known as a geo-heliocentric system, is a hybrid one:

• (i) The earth is motionless at the center of the universe, as in the Ptolemaic, and the moon and sun revolve about it.
  
  
• (ii) But all the other planets (Mercury, Venus, Mars, Jupiter and Saturn) revolve about the sun; in agreement with the Copernican system.

Tycho made an important observation in favor of his system over the Ptolemaic. In 1577 he carefully observed (by naked eye) the passage of a comet, and was able to show that it moved around the sun in an orbit just outside that of Venus; he was also able to observe a very small parallax, consistent with the notion that it was orbiting the sun and not the earth. Indeed, Tycho, unlike Copernicus, made a vast number of observations, and was able to compile a wealth of data, the most important since those of Ptolemy himself. Tycho would bequeath this treasure to his assistant, Kepler, who would go on to develop an elliptical Copernican model.

Some Lesser Known Copernicans: Digges, Gilbert and Bruno

But this is not to say that there were no supporters of Copernicus. In England, for example, at least two interesting figures defended it. Thomas Digges in 1576 issued a book defending a Copernican view, to which he added his own twist: rather than having the stars in a fixed sphere at the periphery, he believed in an infinite universe, with the stars extending without limit beyond the orbit of Saturn. His book, entitled A Perfict Description of the Celestial Orbes, was subtitled as follows: "according to the most ancient doctrine of the Pythagoreans; lately revived by Copernicus and by Geomerical Demonstrations approved."

A scientist of note in another field who approved of at least part of what Copernicus wrote was William Gilbert, whose work de Magnete on the theory of the magnet, with many experiments, was published in 1600. He accepted the rotation of the earth, which he called its "daily magnetic revolution" believing it to be due to magnetic forces, and more reasonable to assume than the opposite assumption of the rotation of the sphere of the stars. Like Digges, he placed the stars at varying distances from the earth outside of the orbit of Saturn, but it is not clear whether he thought they extended without limit. He hesitated between the Copernican and the Tychonic systems. At any rate, he believed that the sun could maintain the planets in their orbits through magnetic emmanations.

In Italy, and indeed throughout Europe because of his wide travels, the Copernican system was defended by Giordana Bruno, especially in his book de Immenso. He believed not only in an infinite universe, but in an infinite number of worlds as well (like the Epicureans before him). "... this world itself was merely one of an infinite number of particular worlds similar to this, and that al lthe planets and other stars are infinite worlds without number composing an infinite universe, so that there is a double infinitude, that of the greatness of the universe, and that of the multitude of worlds." (Stimson, p. 51) His work was not however of a scientific sort, but more polemical. He was most interested in piquing orthodoxy, and he delighted in arguing with learned professors in university debates. He especially liked to contradict Aristotilean professors, which he did with gusto, especially at Oxford and Cambridge when he visited there, and his books contained much reference to false, as opposed to true religion, clearly targeting the established church. But upon one of his returns to Italy, he found that some people didn't like being contradicted. He was arrested by the Inquisition at Venice. He refused to repent, and was burned at the stake at Campo di Fiori, Feb. 17, 1600; his books were placed on the Index of prohibited readings. The Bruno case, incidentally involved more than just Copernicanism. It included his extension of the Copernican view to an infinite model, his attacks on established religion, and his political links with Protestant forces the Vatican disapproved of.

Kepler's Three Laws of Planetary Orbits

But none of the above mentioned writers (Digges, Gilbert, and Bruno) were able to came with Tycho's successor, Johannes Kepler. Tycho Brahe left his many observations, especially of the planet Mars, to his research assistant: Kepler. (The observatory on an island off the coast of Denmark where the observations were made was destroyed by the peasant inhabitants, who especially disliked Tycho for his dictatorial rule over them). Kepler, convinced for philosophical reasons we'll examine later, that a mathematical relation must exist between such variables as a planet's speed of rotation around the sun, its average distance from the sun, and its period of rotation about the sun, developed what later became known as his "three laws of planetary motion.

The first and most radical was that the orbit of a planet (based on data for Mars supplied by Tycho's and his work) was an ellipse, not a circle. This was a second fundamental break with ancient cosmology. The first was Copernicus' replacing of the earth by the sun as center of the universe; now Kepler replaced the circle with the ellipse for the shape of planetary orbits:

(I) Law of ellipses (1609): The orbit of a planet follows the path of an ellipse with the sun at one of its foci. An ellipse is a geometric figure such that the sum of the distances of a point from each of the foci is constant.

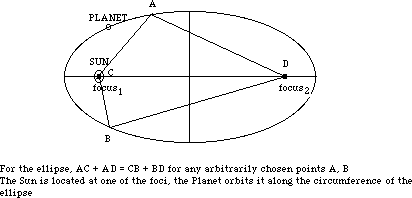
This shift from circle to ellipse was of considerable significance. By more accurately depicting the orbit of a planet, it eliminated the need for epicycles in the Copernican system, one of the principal failings of the project as originally conceived.

At the same time, Kepler determined a crucial quantitative relationship between the velocity of a planet around the sun and its distance from the sun:

(II) Law of Areas (1609): In equal periods of time, a planet sweeps out equal areas with respect to the sun.

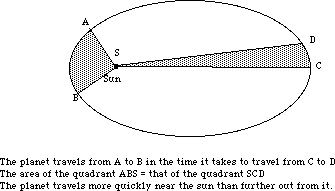
This discovery eliminated the need for equants in order to describe differences in velocity during the orbit of a planet around the sun.

The third great discovery of Kepler was the Law of Proportions according to which the square of the period of revolution of a planet about the sun divided by the cube of its average distance from the sun is a constant. This established an invariant quantitative relationship between two variables, p and d, such that p3/d2 = k, where k is a constant.

(III) Law of Proportions (1615): The square of the period of revolution of a planet about the sun is proportional to the cube of its average distance from the sun.

Planet	Period of rev.P in AU	P2	Avg. dist d in AU	d3	P2/d3
Mercury	0.24	0.06	0.39	0.06	1.00
Venus	0.61	0.37	0.72	0.37	1.00
Earth	1.00	1.00	1.00	1.00	1.00
Mars	1.88	3.53	1.52	3.51	1.01
Jupiter	11.68	136.42	5.20	140.61	0.99
Saturn	29.50	870.25	9.54	868.25	1.00

With Kepler, a simplified system of planetary motions was possible, with for the first time no need for epicycles or any other geometrical hypotheses. Retrogressions of the planets, unequal seasons of the sun, varying velocities of planetary motion and degrees of planetary brightness were all explained in this one very nice system. Note that Kepler largely and almost exclusively based himself on Tycho's and his own observations of Mars, the superior planet closest to the earth.

Kepler's Theological and Philosophical Views/a>